The Fourth Dimension
GA 324a
On Higher-Dimensional Space
22 October 1908, Berlin
Today's subject will present us with a variety of difficulties, and this lecture you requested must be seen as one in a series. A profound understanding of the subject, even on a merely formal level, requires previous mathematical knowledge. Grasping the reality of the subject, however, requires deeper insight into esotericism. We will be able to address this aspect only very superficially today, providing stimulus for further thought. It is very difficult to talk about higher dimensions at all, because in order to picture any dimensions beyond the ordinary three, we must enter abstract realms, where we fall into an abyss if our concepts are not very precisely and strictly formulated. This has been the fate of many people we know, both friends and foes. The concept of higher-dimensional space is not as foreign to mathematics as we generally believe. [Note 67] Mathematicians are already performing calculations involving higher-dimensional operations. Of course, mathematicians can speak about higher-dimensional space only to a very limited extent,—essentially, they can discuss only the possibility that it exists. Determining whether or not such space is real must be left to those who actually can see into it. Here we are dealing with pure concepts that, if precisely formulated, will truly clarify our concept of space.
What is space? We usually say that space is all around us, that we walk around in space, and so on. To gain a clearer idea of space, we must accept a higher level of abstraction. We call the space we move around in three-dimensional. It extends upward and downward, to the right and to the left, and forward and backward. It has length, width, and height. When we look at objects, we see them as occupying three-dimensional space, that is, as possessing a certain length, width, and height. We must deal with the details of the concept of space, however, if we wish to achieve greater clarity. Let's look at the simplest solid shape, the cube, as the clearest example of length, width, and height. The length and width of a cube's lower surface are equal. When we raise this lower surface until its height above its original location is the same as its length or width, we get a cube, that is, a three-dimensional figure. When we examine the boundaries of a cube, we find that they consist of plane surfaces, which are bounded in turn by sides of equal length. A cube has six such plane surfaces.
What is a plane surface? At this point, those incapable of very keen abstractions will begin to go astray. For example, it is impossible to cut off one of the boundaries of a wax cube in the form of a very thin layer of wax, because we would always get a layer with a certain thickness—that is, a solid object. We can never arrive at the boundary of the cube in this way. Its real boundary has only length and width, but no height—that is, no thickness. Thus, we arrive at a formula: a plane surface is one boundary of a three-dimensional figure and has one less dimension. Then what is the boundary of a plane surface such as a square? Again, the definition requires a high degree of abstraction. The boundary of a plane figure is a line, which has only one dimension, length. Width has been eliminated. What is the boundary of a line segment? It is a point, which has zero dimensions. Thus we always eliminate one dimension to find the boundary of a geometric figure.
Let's follow the train of thought of many mathematicians, including Riemann, who has done exceptionally good work. [Note 68] Let's consider a point, which has zero dimensions,—a line, which has one,—a plane, which has two,—and a solid object, which has three. On a purely technical level, mathematicians ask whether it is possible to add a fourth dimension. If so, the boundary of a four-dimensional figure would have to be a three-dimensional figure, just as a plane is the boundary of a solid body, a line the boundary of a plane, and a point the boundary of a line segment. Of course, mathematicians can then proceed to consider figures with five, six, seven, or even ii dimensions, where h is a positive integer.
At this point a certain lack of clarity enters in, when we say that a point has zero dimensions, a line one, a plane two, and a solid object three. We can make solid objects, such as cubes, out of any number of materials—wax, silver, gold, and so on. Their materials are different, but if we make them all the same size, each one occupies the same amount of space. If we then eliminate all the matter these cubes contain, we are left with only specific segments of space, the spatial images of the cubes. These segments of space are the same size for all the cubes, regardless of the material of which they were made, and they all have length, width, and height. We can imagine such cubical spaces extending to infinity, resulting in an infinite three-dimensional space. The material object is only a segment of this space. The next question is, Can we extend our conceptual considerations, which took space as their point of departure, to higher realities? For mathematicians, such considerations include only calculations involving numbers. Is this permissible? As I will now show you, using numbers to calculate the size of spaces results in great confusion. Why is this so? A single example will suffice. Imagine you have a square figure. This plane figure can be made broader and broader on both sides, until eventually we have a plane figure that extends to infinity between two lines (Figure 56)
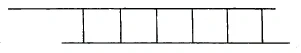
Because this plane figure is infinitely wide, its size is infinity (oo). Now suppose other people hear that the area between these two lines is infinitely large. Naturally, these people think of infinity. But if you mention infinity, they may get a totally incorrect idea of what you mean. Suppose I add another square to each of the existing ones, that is, a second row of infinitely many squares. The result is again infinity, but a different infinity that is exactly twice as great as the first (Figure 57). Consequently, \(∞ = 2\cdot∞\).
In the same way, I could also arrive at \(∞ = 3\cdot∞\). In calculating with numbers, infinity can be used just as easily as any finite number. It is true in the first case that the space is infinite, but it is just as true in the latter instances that it is \(2\cdot∞\), \(3\cdot∞\), and so on. That's what happens when we calculate using numbers.

You see, as long as the concept of infinite space is linked to a numerical reckoning, it makes it impossible to penetrate more deeply into higher realities. Numbers actually have no relationship to space. Like peas or any other objects, numbers are totally neutral with regard to space. As you know, numerical calculations in no way change the reality of the situation. If we have three peas, multiplication cannot change that fact, even if we multiply correctly. Calculating that \(3 \times 3 = 9\) will not produce nine peas. Merely thinking about something changes nothing in such cases, and numerical calculations are mere thinking. We are left with three peas, not nine, even if we performed the multiplication correctly. Similarly, although mathematicians perform calculations pertaining to two, three, four, or five dimensions, the space that confronts us is still three-dimensional. I'm sure you can experience the temptation of such mathematical considerations, but they prove only that it is possible to perform calculations concerning higher-dimensional space. Mathematics cannot prove that higher-dimensional space actually exists,—it cannot prove that the concept is valid in reality. We must be rigorously clear on this point.
Let's consider some of the other very astute thoughts mathematicians have had on this subject. We human beings think, hear, feel, and so on in three-dimensional space. Let's imagine beings capable of perceiving only in two-dimensional space. Their bodily organization would force them to remain in a plane, so they would be unable to leave the second dimension. They would be able to move and perceive only to the right and left and forward and backward. They would have no idea of anything that exists above or below them. [Note 69]
Our situation in three-dimensional space, however, may be similar. Our bodily organization may be so adapted to three dimensions that we cannot perceive the fourth dimension but can only deduce it, just as two-dimensional beings would have to deduce the existence of the third dimension. Mathematicians say that it is indeed possible to think of human beings as being limited in this particular way. Of course, it is certainly possible to say that even though this conclusion might be true, it might also simply be a misinterpretation. Here again, a more exact approach is required, though the issue is not as simple as the first example, where we tried to use numbers to understand the infinity of space. I will deliberately restrict myself to simple explanations today.
The situation with this conclusion is not the same as with the first, purely technical arithmetical line of reasoning. In this instance, there is really something to take hold of. It is true enough that a being might exist who could perceive only objects that move in a plane. Such a being would be totally unaware of anything existing above or below. Imagine that a point within the plane becomes visible to the being. Of course, the point is visible only because it lies within the plane. As long as the point moves within the plane, it remains visible, but as soon as it moves out of the plane, it becomes invisible. It disappears as far as the plane-being is concerned. Now let's suppose that the point appears later somewhere else. It becomes visible again, disappears again, and so on. When the point moves out of the plane, the plane-being cannot follow it but may say, "In the meantime, the point is somewhere where I cannot see it." Let's slip into the mind of the plane-being and consider its two options. On the one hand, it might say, 'There is a third dimension, and that object disappeared into it and later reappeared.” Or it could also say, "Only stupid beings talk about a third dimension. The object simply disappeared, and each time it reappeared, it was created anew." In the latter case, we would have to say that the plane-being violates the laws of reason. If it does not want to assume that the object repeatedly disintegrates and is recreated, it must acknowledge that the object disappears into a space that plane-beings cannot see. When a comet disappears, it passes through four-dimensional space. [Note 70]
Now we see what must be added in a mathematical consideration of this issue. We would have to find something in our field of observation that repeatedly appears and disappears. No clairvoyant abilities are needed. If the plane-being were clairvoyant, it would know from experience that there is a third dimension and would not have to deduce its existence. Something similar is true of human beings. Anyone who is not clairvoyant is forced to say, "I myself am restricted to three dimensions, but as soon as I observe something that disappears and reappears periodically, I am justified in saying that a fourth dimension is involved."
Everything that has been said thus far is completely incontestable, and its confirmation is so simple that it is unlikely to occur to us in our modern state of blindness. The answer to the question, "Does something exist that repeatedly disappears and reappears?" is very easy. Just think of the pleasure that sometimes rises in you and then disappears again, so that no one who is not clairvoyant can still perceive it. Then the same feeling reappears because of some other event. In this case, you, like the plane-being, can behave in one of two ways. Either you can say that the feeling has disappeared into a space where you cannot follow it, or you can insist that the feeling vanishes and is created anew each time it reappears.
It is true, however, that any thought that disappears into the unconscious is evidence of something that can disappear and then reappear. If this idea seems plausible to you, the next step is to attempt to formulate all the possible objections that could be raised from the materialistic viewpoint. I will mention the most pertinent objection now,—all the others are very easy to refute. People may claim to explain this phenomenon in purely materialistic terms. I want to give you an example of something that disappears and reappears in the context of material processes. Imagine a steam piston in action. As long as force is applied to the piston, we perceive its motion. Now suppose we counteract its motion with an identical piston working in the opposite direction. The movement stops and the machines are motionless. The movement disappears.
Similarly, people might claim that the sensation of pleasure is nothing more than molecules moving in the brain. As long as the molecules are moving, I experience pleasure. Let's assume that some other factor causes an opposite movement of molecules. The pleasure disappears. Anyone who does not pursue this line of thought very far might indeed find it a very significant argument against the ideas presented earlier, but let's take a closer look at this objection. Just as the movement of a piston disappears as a result of an opposite movement, a feeling that is based on molecular movement is said to be eliminated by an opposing molecular movement. What happens when one piston movement counteracts another? Both the first and the second movement disappear. The second movement cannot eliminate the first without eliminating itself, too. The result is a total absence of movement,—no movement remains. Thus, no feeling that exists in my consciousness could ever eliminate another without also eliminating itself. The assumption that one feeling can eliminate another is therefore totally false. In that case, no feeling would be left, and a total absence of feeling would result. The most that can still be said is that the first feeling might drive the second into the subconscious. Having said this, however, we admit the existence of something that persists yet evades our direct observation. Today we have been speaking only about purely mathematical ideas, without considering clairvoyant perception at all. Now that we have admitted the possibility that a four-dimensional world exists, we may wonder whether we can observe a four-dimensional object without being clairvoyant. A projection of sorts allows us to do so. We can turn a plane figure until the shadow it casts is a line. Similarly, the shadow of a line can be a point, and the shadow image of a solid three-dimensional object is a two-dimensional plane figure. Thus, once we are convinced of the existence of a fourth dimension, it is only natural to say that three-dimensional figures are the shadow images of four-dimensional figures.

This is one purely geometric way of imagining four-dimensional space. But there is also a different way of visualizing it with the help of geometry. Imagine a square, which has two dimensions. Now picture the four line segments that form its boundaries straightened out to form a single line. You have just straightened out the boundaries of a two-dimensional figure so that they lie in one dimension (Figure 58). Let's take this process one step further. Imagine a line segment. We proceed just as we did with the square, (removing one dimension) so that the boundaries of the figure fall in two points. We have just depicted the boundaries of a one-dimensional figure in zero dimensions. We can also unfold a cube, flattening it into six squares (Figure 59). We unfold the boundaries of a cube so that it lies in a plane. In this way, we can say that a line can be depicted as two points, a square as four line segments, and a cube as six squares. Note the sequence of numbers: two, four, six.

Next we take eight cubes. Just as the previous examples consist of the unfolded boundaries of geometric figures, the eight cubes form the boundaries of a four-dimensional figure (Figure 60). Laying them out results in a double cross that represents the boundaries of a regular four-dimensional figure. Hinton calls this four-dimensional cube a tessaract.
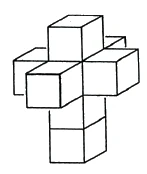
This exercise gives us a mental image of the boundaries of a tessaract. Our idea of this four-dimensional figure is comparable to the idea of a cube that two-dimensional beings can develop by flattening a cube's boundaries, that is, by unfolding them.
The first mathematical studies of the problem of higher-dimensional space date from the middle of the nineteenth century. See the introduction to Manning's Geometry of Four Dimensions [1914],
In the passages that follow, Rudolf Steiner bases himself on Riemann's studies on the geometry of n-dimensional manifolds. See Note 1, Lecture 1 (March 24, 1905).
See also the following books, which were well-known and popular in their time: Abbott, Flatland [1884], Hinton, the chapter "A Plane World" in Scientific Romances [1886] (pp. 129-159), and Hinton, An Episode of Flatland [1907],
See also Rudolf Steiner's lecture of April 10, 1912 (GA 136). We have not been able to confirm the assumption that this statement of Steiner's refers to Zöllner’s views on the subject. Zöllner's comet theory (see Zöllner [1886]) became the basis and point of departure for modern conventional comet theories, and there is no indication that Zöllner saw any connection between his comet theory and his spiritualistic ideas about four-dimensional space.

