The Fourth Dimension
GA 324a
First Lecture
24 March 1905, Berlin
Because I will begin by discussing elementary aspects of the fourth dimension, what you hear today may disappoint you, but dealing with these issues in greater depth would require a thorough knowledge of the concepts of higher mathematics. I would first like to provide you with very general and elementary concepts. We must distinguish between the reality of four-dimensional space and the possibility of thinking about it. Four-dimensional space deals with a reality that goes far beyond ordinary sense-perceptible reality. When we enter that realm, we must transform our thinking and become familiar with the way in which mathematicians think.
We must realize that at each step mathematicians take, they must account for its impact on their entire line of reasoning. When we concern ourselves with mathematics, however, we also must realize that even mathematicians cannot take a single step into four-dimensional reality. [They can arrive at conclusions only from what can and cannot be thought.]. The subjects we will deal with are initially simple but become more complicated as we approach the concept of the fourth dimension. We first must be clear about what we mean by dimensions. The best way to gain clarity is to check the dimensionality of various geometrical objects, which then will lead us to considerations that were first tackled in the nineteenth century by such great mathematicians as Bolyai, Gauss, and Riemann. [Note 01]
The simplest geometrical object is the point. It has no size,—it can only be imagined. It fixes a location in space. It has a dimension which equals zero. The first dimension is given by a line. A straight line has one dimension,—length. When we move a line, which has no thickness, it leaves the first dimension and becomes a plane. A plane has two dimensions,—length and breadth. When we move a plane, it leaves these two dimensions. The result is a solid body with three dimensions—height, breadth, and depth (Figure 1).

When you move a solid body (such as a cube) around in space, however, the result is still only a three-dimensional body. You cannot make it leave three-dimensional space by moving it. There are still a few more concepts we need to look at. Consider a straight line segment. It has two boundaries, two endpoints—point \(A\) and point \(B\) (Figure 2).

Suppose we want to make point \(A\) and point \(B\) meet. To do this, we must bend the straight line segment. What happens then? It is impossible to make points \(A\) and \(B\) coincide if you stay within the [one-dimensional] straight line. To unite these two points, we must leave the straight line—that is, the first dimension—and enter the second dimension, the plane. When we make its endpoints coincide, the straight line segment becomes a closed curve, that is, in the simplest instance, a circle (Figure 3).

A line segment can be transformed into a circle only by leaving the first dimension. You can duplicate this process with a rectangular surface, but only if you do not remain in two dimensions. To transform the rectangle into a cylinder or tube, you must enter the third dimension. This operation is performed in exactly the same way as the preceding one, in which we brought two points together by leaving the first dimension. In the case of a rectangle, which lies in a plane, we must move into the third dimension in order to make two of its boundaries coincide (Figure 4).

Is it conceivable to carry out a similar operation with an object that already has three dimensions? Think of two congruent cubes as the boundaries of a three-dimensional rectangular solid. You can slide one of these cubes into the other. Now imagine that one cube is red on one side and blue on the opposite side. The only way to make this cube coincide with the other one, which is geometrically identical but whose red and blue sides are reversed, would be to turn one of the cubes around and then slide them together (Figure 5).

Let's consider another three-dimensional object. You cannot put a left-handed glove onto your right hand. But if you imagine a pair of gloves, which are symmetrical mirror images of each other and then you consider the straight line segment with its endpoints \(A\) and \(B\), you can see how the gloves belong together. They form a single three-dimensional figure with a boundary, (the mirroring plane), in the middle. The same is true of the two symmetrical halves of a person's outer skin. [Note 02] How can two three-dimensional objects that are mirror images of each other be made to coincide? Only by leaving the third dimension, just as we left the first and second dimensions in the previous examples. A right- or left-handed glove can be pulled over the left or right hand, respectively, by going through four-dimensional space. [Note 03] In building up depth, the third dimension of perceived space, we pull the image from our right eye over the image from our left eye, that is, we fuse the two images. [Note 04]
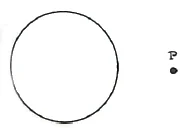
Now let's consider one of Zöllner's examples. [Note 05] Here we have a circle and, outside it, a point \(P\) (Figure 6). How can we bring point \(P\) into the circle without cutting the circumference? We cannot do this if we remain within the plane. Just as we need to leave the second dimension and enter the third in order to make the transition from a square to a cube, we must also leave the second dimension in this example. Similarly, in the case of a sphere, it is impossible to get to the interior without either piercing the sphere's surface or leaving the third dimension. [Note 06]
These are conceptual possibilities, but they are of immediate practical significance to epistemology, especially with regard to the epistemological problem of the objectivity of the contents of perception. We first must understand clearly how we actually perceive. How do we acquire knowledge about objects through our senses? We see a color. Without eyes we would not perceive it. Physicists tell us that what is out there in space is not color but purely spatial movement patterns that enter the eye and are then picked up by the visual nerve and conveyed to the brain, where the perception of the color red, for example, comes about. Next, we may wonder whether the color red is present when sensation is not.
We could not perceive red if we had no eyes or the sound of bells ringing if we had no ears. All of our sensations depend on movement patterns that are transformed by our psycho-physical apparatus. The issue becomes even more complicated, however, when we ask where that unique quality "red" is located—is it on the object we perceive, or is it a vibrational process? A set of movements that originates outside us enters the eye and continues into the brain itself. Wherever you look, you find vibrational processes and nerve processes, but not the color red. You also will not find it by studying the eye itself. It is neither outside us nor in the brain. Red exists only when we ourselves, as subjects, intercept these movements. Is it impossible then to talk about how red comes to meet the eye or C-sharp the ear?
The questions are, what is an internal mental image of this sort, and where does it arise? These questions pervade all of nineteenth- century philosophy. Schopenhauer proposed the definition "The world is our mental image." [Note 07] But in this case, what is left for the external object? Just as a mental image of color can be "created" by movement, so, too, the perception of movement can come about within us as a result of something that is not moving. Suppose we glue twelve snapshots of a horse in motion to the inner surface of a cylinder equipped with twelve narrow slits between the images. When we look sideways at the turning cylinder, we get the impression that we are always seeing the same horse and that its feet are moving. [Note 08] Our bodily organization can induce the impression of movement when the object in question is really not moving at all. In this way, what we call movement dissolves into nothing.
In that case, what is matter? If we strip matter of color, movement, shape, and all other qualities conveyed through sensory perception, nothing is left. If "subjective" sensations, such as color, sound, warmth, taste, and smell, which arise in the consciousness of individuals as a result of environmental stimuli, must be sought within ourselves, so, too, must the primary, "objective" sensations of shape and movement. The outer world vanishes completely. This state of affairs causes grave difficulties for epistemology. [Note 09]
Assuming that all qualities of objects exist outside us, how do they enter us? Where is the point at which the outer is transformed into the inner? If we strip the outer world of all the contents of sensory perception, it no longer exists. Epistemology begins to look like Münchhausen trying to pull himself up by his bootstraps. [Note 10] To explain sensations that arise within us, we must assume that the outer world exists, but how do aspects of this outer world get inside us and appear in the form of mental images?
This question needs to be formulated differently. Let's consider several analogies that are necessary for discovering the connection between the outer world and internal sensation. Let's go back to the straight line segment with its endpoints \(A\) and \(B\). To make these endpoints coincide, we must move beyond the first dimension and bend the line (Figure 7).

Now imagine that we make the left endpoint \(A\) of this straight line segment coincide with the right endpoint \(B\) in such a way that they meet below the original line. We can then pass through the overlapping endpoints and return to our starting point. If the original line segment is short, the resulting circle is small, but if I bend ever longer line segments into circles, the point where their endpoints meet moves farther and farther away from the original line until it is infinitely distant. The curvature becomes increasingly slight, until finally the naked eye can no longer distinguish the circumference of the circle from the straight line (Figure 8).

Similarly, when we walk on the Earth, it appears to be a straight, flat surface, though it is actually round. When we imagine the two halves of the straight line segment extended to infinity, the circle really does coincide with the straight line. [Note 11] Thus a straight line can be interpreted as a circle whose diameter is infinitely large. Now we can imagine that if we move ever farther along the straight line, we will eventually pass through infinity and come back from the other side.
Instead of a geometric line, envision a situation that we can associate with reality. Let's imagine that point \(C\) becomes progressively cooler as it moves along the circumference of the circle and becomes increasingly distant from its starting point. When it passes the lower boundary \(A\), \(B\) and begins the return trip on the other side, the temperature starts to rise (Figure 9). Thus, on its return trip, point \(C\) encounters conditions that are opposite to the ones it encountered on the first half of its journey. The warming trend continues until the original temperature is reached. This process remains the same no matter how large the circle,—warmth initially decreases and then increases again. With regard to a line that stretches to infinity, the temperature decreases on one side and increases on the other. This is an example of how we bring life and movement into the world and begin to understand the world in a higher sense. Here we have two mutually dependent activities. As far as sensory observation is concerned, the process that moves to the right has nothing to do with the process that returns from the left, and yet the two are mutually dependent. [Note 12]

Now let's relate the objects of the outer world to the cooling stage and our internal sensations to the warming stage. Although the outer world and our internal sensations are not linked directly by anything perceptible to the senses, they are interrelated and interdependent in the same way as the processes I just described. In support of their interrelationship, we also can apply the metaphor of seal and sealing wax. The seal leaves an exact impression, or copy, of itself in the sealing wax even though it does not remain in contact with the wax and there is no transfer of substance between them. The sealing wax retains a faithful impression of the seal. The connection between the outer world and our internal sensations is similar. Only the essential aspect is transmitted. One set of circumstances determines the other, but no transfer of substance occurs. [Note 13]
Viewing the connection between the outer world and our own impressions in this way, we realize that geometric mirror images in space are like right- and left-handed gloves. To make them coincide directly with a continuous motion, we need the help of a new dimension of space. If the relationship between the outer world and an internal impression is analogous to the relationship between figures that are geometric mirror images, the outer world and the internal impression also can be made to coincide directly only by means of an additional dimension. To establish a connection between the outer world and internal impressions, we must pass through a fourth dimension where we are still in the third. Only there, where we are united with the outer world and inner impressions, can we discover their commonalties. We can imagine mirror images floating in a sea in which they can be made to coincide. Thus we arrive, though initially purely on the level of thinking, at something that is real but transcends three-dimensional space. To do this, we need to enliven our ideas of space.
Oskar Simony attempted to use models to depict enlivened spatial formations. [Note 14] As we have seen, we can move step by step from considering zero dimension to imagining four-dimensional space. Four-dimensional space can be recognized most easily with the help of mirror-image figures or symmetrical relationships. Knotted curves and two-dimensional strips offer another method of studying the unique qualities of empirical, three-dimensional space as it relates to four-dimensional space. What do we mean by symmetrical relationships? When we interlink spatial figures, certain complications arise. These complications are unique to three-dimensional space,—they do not occur in four-dimensional space. [Note 15]
Let's try a few practical thought exercises. When we cut along the middle of a cylindrical ring, we get two such rings. If we give a strip a 180° twist before gluing its ends, cutting it down the middle results in a single twisted ring that will not come apart. If we give the strip a 360° twist before gluing its ends, the ring falls apart into two twisted, interlocking rings when we cut it. And finally, if we give the strip a 720° twist, cutting it results in a knot. [Note 16] Anyone who thinks about natural processes knows that such twists occur in nature. In reality, all such twisted spatial formations possess specific forces. Take, for example, the movement of the Earth around the Sun and then the movement of the Moon around the Earth. We say that the Moon describes a circle around the Earth, but, if we look more closely, we realize that it actually describes a line that is twisted around the circle of the Earths orbit—that is, a spiral around a circle. And then we also have the Sun, which moves so quickly through space that the Moon makes an additional spiral movement around it. Thus, the force-lines extending through space are very complex. We must realize that we are dealing with complicated spatial concepts that we can understand only if we do not try to pin them down but instead allow them to remain fluid.
Let's review what we discussed today. Zero dimension is the point, the first dimension is the line, the second dimension is the surface, and the third dimension is the solid body. How do these spatial concepts relate to one another? Imagine that you are a being who can move only along a straight line. What kind of spatial images do one-dimensional beings have? Such beings would be able to perceive only points, and not their own one-dimensionality, because when we attempt to draw something within a line, points are the only option. A two-dimensional being would be able to encounter lines and thus to distinguish one-dimensional beings. A three-dimensional being, such as a cube, would perceive two-dimensional beings. Human beings, however, can perceive three dimensions. If we draw the right conclusions, we must say that just as a one-dimensional being can perceive only points, a two-dimensional being only one dimension, and a three-dimensional being only two dimensions, a being that perceives three dimensions must be a four-dimensional being. Because we can delineate external beings in three dimensions and manipulate three-dimensional spaces, we must be four-dimensional beings. [Note 17] Just as a cube can perceive only two dimensions and not its own third dimension, it is also true that we human beings cannot perceive the fourth dimension in which we live.
János (Johann) Bólyai (1802-1860), Hungarian mathematician. He studied the problem of parallel lines and, along with Carl Friedrich Gauss and Nikolai Ivanovich Lobachevsky, is considered one of the founders of hyperbolic non-Euclidean geometry. His paper on this subject, his only published work, appeared in 1832 as an appendix to the mathematics text written by his father, Farkas (Wolfyang) Bólyai (1775-1856). For more information on the two Bólyais, see Stäckel [1913].
Carl Friedrich Gauss (1777-1855), mathematician and physicist in Göttingen. One of the first to consider the problem of parallel lines, he concluded that explaining them required a non-Euclidean geometry. None of his work on this subject was published during his lifetime. See Reichardt [1976].
Bernhard Riemann (1826-1866), mathematician in Göttingen and the first to discover elliptical non-Euclidean geometry. His thesis on The Hypotheses Underlying Geometry developed differential geometry by generalized measurements in n-dimensional space. This supplied an incentive for research (then in its infancy) into higher-dimensional space. Riemann was the first to distinguish between limitlessness and infinity of space,—the former is an expression of spatial relationships, that is, of the general geometric structure (topology) of space, while the latter is a consequence of numerical relationships. This distinction led to the clear differentiation between topology and differential geometry. See Scholz [1980].
Immanuel Kant drew attention to this phenomenon in his Prolegomena [1783), §13: "What can be more similar, in all its parts, to my hand or my ear than its image in a mirror? And yet I cannot replace the original with what I see in the mirror, because if the original is a right hand, its mirror image is a left hand, and the image of a right ear is a left ear and can never take the place of its original. There are no intrinsic, rationally conceivable differences between them, and yet our senses teach us that they are indeed intrinsically different, because in spite of all apparent similarity and sameness, a left hand is not contained within the same boundaries as a right hand (that is, they are not congruent) and a glove that fits one hand cannot be worn on the other." See also Kant's Lebendige Kräfte ("Living Forces") [ 1746], §§9-11, and Gegenden im Raum (“Areas in Space") [1768], Kant took this phenomenon as proof that human beings are capable of grasping only sensory perceptions of objects—that is, their appearances—and not their intrinsic nature. For an analysis of Kant's view of space with regard to the dimension problem, see Zöllner, Wirkungen in die Ferne ("Distant Effects") [1878a], pp. 220-227.
- Mirror-image figures that lie in the same plane and meet in an axis can be made to coincide by a continuous motion with each other by rotating one of the figures around the axis. If \(F\) is a figure in the plane and \(F’\) its mirror image on the other side of axis \(a\), \(F\) can be transformed into \(F’\) by rotation around \(a\). Figure 68 shows several stages in this rotation in normal projection onto the plane. The transformation, if interpreted as a plane figure, involves a projection orthogonal to \(a\). (In the sense of projective geometry, this is a perspective with its axis a and center A on the line at infinity of the plane.)

In its projection onto the plane, the figure rotated through space appears to lose a dimension as it passes through axis a and becomes parallel to the direction of projection. Note that the outlines of F and F' can be made to coincide through rotation within the plane (i.e., around points in the plane) only if they are broken into line segments that are then rotated around the corresponding points on axis \(a\).
In an analogous operation, the two three-dimensional geometric figures \(F\) and \(F’\), which are mirror images joined by plane a, can be transformed into each other without breaking contact by means of the (three-dimensional) spatial orthogonal affinity with a as the plane of affinity (Figure 69). This transformation can be interpreted as an orthogonal projection (in three-dimensional space) of a four-dimensional Euclidean rotation around plane a. In this projection, the three-dimensional figure \(F\) seems to lose a dimension as it passes through the two-dimensional plane \(a\).

If the outer surface of \(F\) is broken into appropriate sections, these sections can be rotated around the corresponding axes in a to form the outer surface of figure \(F’\).
Basing his theories on this analogy between two- and three-dimensional mirror images, August Ferdinand Möbius was apparently the first mathematician to conceive of the possibility of a four-dimensional space in which three-dimensional mirror-image figures can be made to coincide without breaking contact (see Möbius's Barycentric Calculus [1827], §140, note). He rejected this idea as "impossible to think," however, and did not pursue it further.
The fact that we have two eyes makes depth perception possible for us; see also Rudolf Steiner's answers to questions by A. Strakosch, March 11, 1920, reprinted in this volume. On the significance of independent activity in perceiving the dimension of depth, see the questions and answers of April 7, 1921 (GA 76, reprinted here), and Note 17 here.
(Johann Karl) Friedrich Zöllner (1834-1882), astrophysicist in Leipzig, considered one of the founding fathers of astrophysics because of his fundamental experimental and theoretical contributions to photometry and spectroscopy. His theory on the structure of comets set the direction for all later investigations. His book On the Nature of Comets: Contributions to the History and Theory of Knowledge [1886], like almost all of his treatises, contains far-reaching philosophical and historical commentary as well as polemical critiques of his contemporaries' pursuit of science.
In connection with his studies on the Principles of an Electrodynamic Theory of Matter [ 1876], On Distant Effects [1878a], and On the Nature of Comets [1886], Zöllner became familiar with contemporary studies of non-Euclidean and higher-dimensional geometry. By the early 1870s, he surmised that only curved space or a fourth dimension could explain certain phenomena of physics. Around 1875, the research of the chemist and physicist William Crookes (1832-1919) inspired Zöllner to study spiritualism. He developed the view that the existence of spiritualistic phenomena could be explained by assuming the existence of four-dimensional space and that these phenomena proved that four-dimensional space is a reality, not merely a conceptual possibility (Zöllner [1878a], pp. 273ff). A short time later, Zöllner began his own studies of spiritualistic phenomena (see [1878b], pp. 752ff; [1878c], pp. 330ff; and especially [1878c]). For an overview of Zöllner's spiritualistic experiments, see Luttenberger [1977], for a contemporary analysis of Zöllner, see Simony's Spiritualistic Manifestations [1884]. On spiritualism in general, see Hartmann's Spirit Hypothesis [1891] and Spiritualism [1898]. On the history of spiritualism from Rudolf Steiner's point of view, see his lectures of February 1 and May 30, 1904 (GA 52), and October 10-25, 1915 (GA 254). Zöllner conceived of Kant's "things as such" as real four-dimensional objects projected into our perceptual space as three-dimensional bodies. He found proof of this view in the existence of three-dimensional mirror-image figures, which, though mathematically congruent, cannot be made to coincide without breaking contact with each other [in three dimensions] (see Note 3): "In fact, space that can explain the world we see without contradictions must possess at least four dimensions, without which the actual existence of symmetrical figures can never be traced back to a [single] law."(Zöllner [1878a], p. 248). Zöllner saw Kant's ideas as a precursor to his own views (see Note 2).
In the essay quoted, Zöllner describes some of the unique characteristics of the transition from the third to the fourth dimension. Both his theoretical considerations and his spiritualistic experiments are based on these characteristics. He begins with a discussion of knots in three-dimensional space and draws attention to the fact that they can be untied only if "portions of the string temporarily disappear from three-dimensional space as far as beings of the same dimensionality are concerned [see Note 15], The same thing would happen if, by means of a movement executed in the fourth dimension, a body were removed from within a completely enclosed three-dimensional space and relocated outside it. Thus it seems possible to nullify the law of the so-called impermeability of matter in three-dimensional space in a manner completely analogous to removing an object from within a closed curve contained in a plane by lifting the object over the boundary of the curve without touching it." (Zöllner [1878a], p. 276.) See also Note 6.
A perpendicular can be dropped to any point on a two-dimensional surface. If a point \(P\) moves away from the surface along this perpendicular, it distances itself from all points on the surface without changing its vertical projection \(M\) on the surface in any way. If this point \(M\) is the midpoint \(M\) of a circle, as point \(P\) leaves the surface, it is always equidistant from any of the points on the periphery of the circle, though this distance is constantly increasing. If we let point \(P\) move out along the perpendicular until its distance from midpoint \(M\) of the circle is greater than the radius of the circle and then rotate the perpendicular until it coincides with the plane of the circle, point \(P\) will have moved out of the circle without cutting through its circumference.
Analogously, a point P inside a sphere can move out of the interior of the sphere without piercing its surface as soon as we enlist the help of four-dimensional space. Any point in three-dimensional space can leave it and enter four-dimensional space along the straight line of a perpendicular without touching any point in the original space. If we remove the midpoint \(M\) of a sphere from three-dimensional space in this way, point \(M\) distances itself increasingly but equally from all points on the sphere's surface. As soon as the distance from the initial location \(M\) is greater than the radius of the sphere, the point has been removed from the sphere, and the operation can be made visible by rotating the straight line along which the point traveled back into three-dimensional space.
Arthur Schopenhauer (1788-1869): "The world is my mental image': this is a truth that applies to any living, cognizant being." (The World as Will and Mental Image, vol. I, §1 [1894], p. 29).
Rudolf Steiner also uses this example in his book Intuitive Thinking as a Spiritual Path: A Philosophy of Freedom (GA 4), chapter VI, "The Human Individuality," p. 106. See also his lecture of January 14, 1921 (GA 323, p. 252).
Rudolf Steiner discusses these difficulties in greater detail in Intuitive Thinking as a Spiritual Path—A Philosophy of Freedom (GA 4), chapter IV, "The World as Perception," and in his Introduction to Goethe's Natural Scientific Works (GA 1), chapter IX, "Goethe's Epistemology," and chapter XVI.2, 'The Archetypal Phenomenon."
Rudolf Steiner also uses this comparison in his lecture of November 8, 1908 (GA 108), where he investigates more closely how sensation, perception, mental images, and concepts relate to each other.
Strictly speaking, this statement about the transition from circle to straight line is valid only in Euclidean geometry. In projective geometry, the transitional circle coincides with both the tangent, which remains constant, and the infinitely distant straight line (see Locher [1937], chapter IV, especially pp. 69ff). Only when the Euclidean plane becomes a projective plane by incorporating the infinitely distant straight line is it possible to pass through infinity (see also Ziegler [1992], chapter III).
This phenomenon is directly related to the geometric fact that it is impossible to pass through infinity without leaving the domain of Euclidean geometry (see Note 11). In other words, the point we imagine as moving in one direction is not transformed into the point we imagine as coming back from the other side. The two portions of the straight line that we can imagine in sensory terms are connected through infinity only by a lawfulness that we can conceive,—they are separated by their manifestation in points that we can visualize.
Rudolf Steiner uses the metaphor of seal, sealing wax, and impression repeatedly in epistemological considerations about the relationship between the objective outer world and the consciousness of the cognizant individual. The decisive aspect of this metaphor is that in it, as in the psycho-physical domain, transmission of form is not bound to transmission of substance. See also Steiners essays Philosophy and Anthroposophy (GA 35) and Anthroposophy's Psychological Foundations and Epistemological Position (GA 35), p. 138.
Oskar Simony (1852-1915), mathematician and scientist in Vienna, son of the geographer and alpine researcher Friedrich Simony (1812-1896) and professor at the Vienna College of Agriculture from 1880 to 1913. His mathematical studies focused on number theory and the empirical and experimental topology of knots and two-dimensional surfaces in three-dimensional space (see Muller [1931] and [1951]). Some of the models Steiner mentions are illustrated in Simony's treatises.
Simony's early involvement with topology was inspired by his encounters with Zöllner's spiritualistic experiments (see Note 5). He felt compelled to study the spatial problems posed by the discovery of non-Euclidean and multidimensional geometry. His investigations expanded to include physiological and epistemological considerations (see Simony [1883], [1884], and [1886]). The importance of not confusing the empirical realm and the realm of mathematical ideas was clear to him. The conceptual possibility of four-dimensional space was not a problem to him as a mathematician, but he could not accept Zöllner's thesis that all objects in three-dimensional space are projections of four-dimensional objects that are not perceptible to the senses. His intention, however, was not to reject the existence of spiritualistic phenomena out of hand. On the contrary, he, like Zöllner, advocated exact scientific investigation of such phenomena. He also considered how the spiritualistic phenomena reported by Zöllner might be proved using the traditional methods of physics and physiology, or at least reconciled with these fields (Simony, Spiritualistic Manifestations [1884], He felt that it was important to demonstrate that explaining such phenomena did not require leaving three-dimensional, empirical space. He pointed out that Zöllner's hypothesis of the existence of four-dimensional space contradicted our ordinary experience of space,—If this hypothesis is correct, objects in the ordinary three-dimensional space of physics are shadow images that we can change at will without having direct access to their prototypes (Simony [1881b], §6, and [1884], pp. 20ff). As shown by the example of a shadow projected by a three-dimensional object onto a surface, however, no change in the shadow is possible without direct access to the object that casts it.
Simony's topological experiments were intended to investigate the nature of three-dimensional, empirical space, as opposed to curved space or any other mathematically conceivable space: 'The phenomena investigated here, since they belong to the realm of our senses, [can] be incorporated only into an empirical geometry without being brought into connection with the theory of so-called higher manifolds. In addition, the course of development I chose also makes it clear why, in investigating various sections of the first and second type, I avoided using either analytical geometry or infinitesimal calculus in order to remain independent of any possible hypothesis about the nature of perceived space" ([1883], pp. 963ff).
As a mathematician, Simony was especially interested in how knots develop in twisted ring-shaped surfaces and in unknotted cross-shaped closed surfaces. He demonstrated that such surfaces can be cut in ways that either do not destroy their closed character or produce knots, under appropriate circumstances (Simony [1880], [1881a], [1881b]. The simplest and most famous example of this type, a closed strip incorporating a 720° twist, is mentioned by Rudolf Steiner in this lecture.
In four-dimensional space, there are no knots,—that is, every knot in a closed thread or strip can be untied simply by pulling, without cutting (opening) the thread or strip.
Felix Klein (1845-1925) seems to have been the first mathematician to draw attention to this phenomenon in the early 1870s. According to an account by Zöllner [1878a], Klein spoke with him during a scientific conference on this subject shortly before publishing a treatise [1876] in which he discussed this theme in passing. Klein also reported on their meeting and expressed the opinion that it inspired Zöllner's thesis on the existence of four-dimensional space and its significance in explaining spiritualistic phenomena (Klein [1926], pp. 169ff). While Klein ([1876], p. 478) discusses the subject only in general terms, Hoppe [1879] uses an analytically formulated example to untie concretely a simple three-dimensional knot in four-dimensional space (see also Durège [1880] and Hoppe [1880]).
In Distant Effects ([1878a], pp. 272-274), Zöllner demonstrates the dissolution of knots in four-dimensional space with the help of an analogy. He first considers the dissolution of a two-dimensional knot in a closed curve (Figure 70): Without cutting the curve, the crossing cannot be eliminated if we remain within the plane, but by rotating a section of the curve through three-dimensional space around a straight line lying in the plane, any crossing can be undone without cutting the curve.
"If these considerations are transferred via analogy to a knot in three-dimensional space, it is easy to see that such a knot can be tied and untied only through operations in which the elements of the thread describe a doubly bent curve." Without being cut, this knot cannot be untied in three-dimensional space. "If, however, there were beings among us capable of carrying out fourdimensional movements of material objects, these beings would be able to tie and untie such knots much faster, by means of an operation fully analogous to untying the two-dimensional knot described above. [...] My observations on knot formation in a flexible thread in different dimensions of space were inspired by oral communications from Dr. Felix Klein, professor of mathematics in Munich.
"Clearly, in the operations indicated here, portions of the thread must disappear temporarily from three-dimensional space, as far as beings of the same dimensionality are concerned" (Zöllner [1878a], pp. 273-276).
Undoing a knot in three-dimensional space is indeed always possible if either self-crossing or passing through four-dimensional space is allowed, since the latter makes possible the results of self-crossing without the actual self-crossing (see Seifert/Threlfall [1934], p. 3 and p. 315). All we need to do is rotate a suitably shaped section of the curve in plane a around plane b through four-dimensional space (Figure 71).Giving a strip a 360° twist before joining its ends into a ring results in a surface that is the four-dimensional equivalent of a three-dimensional cylindrical ring (Figure 72).
In other words, twists that are whole-number multiples of 360° can be undone in four-dimensional space (see later discussion). Simony was presumably aware of this phenomenon, though he does not mention it explicitly in his topological works, since he was primarily concerned with the unique qualities of empirical three-dimensional space. The equivalence of an untwisted cylindrical strip in three-dimensional space and a strip with a 360° twist in four-dimensional space results from the fact that both rings are characterized by two non-intersecting curved edges. In the second instance, these curved edges are twisted around each other, while in the first instance they are not. In four-dimensional space, the twisting can be undone without any overlapping, converting the twisted ring into an untwisted ring (see the transition from Figure 73 to 74).
Note that this operation cannot be performed on the so-called Möbius strip, a cylindrical ring incorporating a 180° twist (Figure 75). This surface has only one edge curve,—even in four-dimensional space, it cannot be transformed into an untwisted ring in any way without cutting through the surface. (This phenomenon has to do with the fact that such a surface cannot be oriented,—see Seifert/Threlfall [1934], §2. The Möbius strip was first described by Möbius [1865], §11.)
Geometrically speaking, (static) vision in a plane or in space can be interpreted as a central projection of objects in the plane or in space onto a surface. To a being in three-dimensional space with this type of vision, therefore, all objects would appear as if projected on a surface. This being has an indirect impression of the third dimension only if it is able to see dynamically; that is, if its visual apparatus includes two projection directions and the ability to accommodate them. If not, such a being would be able to conclude that the third dimension exists (as one-eyed people do on the basis of much experience and many opportunities for comparison) but would not be able to experience it. The very fact of three-dimensional dynamic vision in human beings is evidence of our "four-dimensional" nature, which we cannot perceive directly (i.e. by means of our senses), though we can conclude that it exists.
On the basis of geometry and physics, Charles Howard Hinton (1853-1907) also concluded that human beings must be beings of four or more dimensions. "It can be argued that symmetry in any number of dimensions is the evidence of an action in a higher dimensionality. Thus considering living beings, there is evidence both in their structure, and in their different mode of activity, of a something coming in from without into the inorganic world." (Hinton, The Fourth Dimension [1904], p. 78).

